
My latest posts can be found here: Previous blog posts:
Additionally, some earlier writings: |
There's an old story that goes something like this:
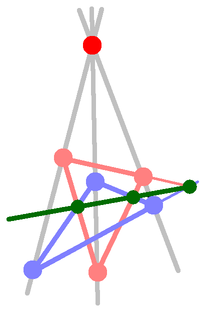
Clearly (for some definition of the word) we are expected to find it amusing that it takes many hours of work and many pages of working to discover that something is "obvious", and yet the word and its ilk persist in the literature. "Clearly" this, "obviously" that, and no, it's not at all obvious to me. All you're doing is making me feel stupid. But that's not the intent. At least, we would hope it's not the intent, and it certainly is not the intent when I use it. So what is the intent? What does it really mean? There is a way of thinking about "clearly" and its relatives that makes it clear that the word is serving a positive purpose. It goes like this. Let's suppose that the author does, in truth, find this obvious, and yet I don't. What does that tell me? It tells me that I'm not looking at it the right way, or from the right point of view. Here's a theorem:
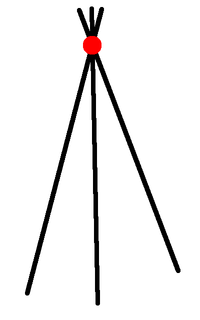
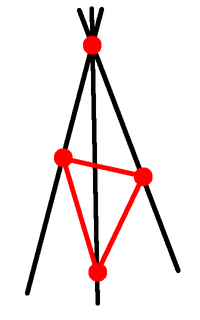
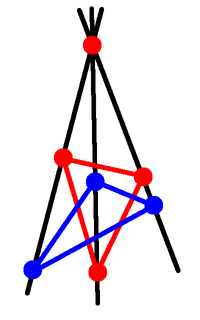
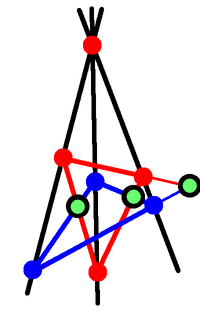
The way to see it is to pull the middle line out of the page so the original three lines form a tripod. Then think of each triangle as the intersection between a plane and the tripod. The two planes meet in a line, and the intersections between the corresponding sides are on that line, and once you see it, it's nearly impossible to unsee it. It becomes obvious, once you think about it the right way, and stand in the right place.
When an author writes "clearly" they are saying "Once you are thinking about this the right way, this is obvious. If it's not (yet) obvious, you are not (yet) in the right frame of mind to progress. You need to change how you're thinking about it. Read on, by all means, but it might be worth working on this bit for a while to see if you get the insight necessary to make this obvious, and that will help to make what follows obvious too." OK, maybe they don't always mean that. Maybe sometimes they really are saying "I'm cleverer than you," but if you use this as a coded message to say "This bit requires some work to get into the right frame of mind," then you can turn it into something constructive.
CommentsI've decided no longer to include comments directly via the Disqus (or any other) system. Instead, I'd be more than delighted to get emails from people who wish to make comments or engage in discussion. Comments will then be integrated into the page as and when they are appropriate. If the number of emails/comments gets too large to handle then I might return to a semi-automated system. That's looking increasingly unlikely.
|
 Suggest a change ( <--
What does this mean?) /
Send me email
Suggest a change ( <--
What does this mean?) /
Send me email