Subscribe!

• Colins Blog

• One Of My Favourite Puzzles
• Thinking About Recursion
• Memorising The Tube
• Spikey Spheres
• Surprisingly Quick
• An Unexpected Fraction
• You Have To Admire Their Optimism
• Representatives Matter
• Pythagoras By Incircle
• A Puzzle About Puzzles
• How Not To Do Twitter
• Calculating 52 Factorial By Hand
• Small Things Might Not Be So Small
• Not If You Hurry
• Factoring Via Graph Three Colouring
• Another Proof Of The Doodle Theorem
• When Obvious Is Not Obvious
• Graph Three Colouring
• The Doodle Theorem
• Be Careful What You Say
• The Mutilated Chessboard Revisited
• A Mirror Copied
• The Other Other Rope Around The Earth
• Photocopy A Mirror
• The Point Of The Banach Tarski Theorem
• Sieve Of Eratosthenes In Python
• Fast Perrin Test
• Russian Peasant Multiplication
• FindingPerrinPseudoPrimes Part2
• FindingPerrinPseudoPrimes Part1
• The Unwise Update
• Miles Per Gallon
• Tracking An Item On Hacker News
• Hacker News User Ages
• Poking The Dusty Corners
• There Is No Time For This
• Publically Sharing Links
• Learning Times Tables
• Graceful Degradation
• Diagramming Maths Topics
• On The Rack
• Square Root By Long Division
• Beyond The Boundary
• Fill In The Gaps
• Software Checklist
• NASA Space Crews
• The Birthday Paradox
• The Trapezium Conundrum
• Revisiting The Ant
• The Ant And The Rubber Band
• Irrationals Exist
• Multiple Choice Probability Puzzle
• Random Eratosthenes
• Wrapping Up Square Dissection
• Dissecting A Square Part 2
• Dissecting A Circle
• Dissecting A Square
• An Oddity In Tennis
• Decision Tree For Tennis
• Decision Trees In Games
• A Matter Of Convention
• Do You Nourish Or Tarnish
• Binary Search Reconsidered
• Two Equals Four
• The Lost Property Office
• The Forgiving User Interface
• Setting Up RSS
• Withdrawing From Hacker News

• Random Writings.
• Colins Blog 2010
• Colins Blog 2009
• Colins Blog 2008
• Colins Blog 2007
• Colins Blog Before 2007

The Independence Game - 2017/04/16

This page has been Tagged As Maths.

A few weeks ago I was honoured to be able to attend the gathering at the Royal Society where Rob Eastaway was given his Christopher Zeeman medal. He gave an excellent talk, full of interest and humour, the only downside being that he made us play a game. I don't remember if he gave it a name (Update: Rob calls it "Avoid the Neighbours") but I now think of it simply as "Rob's Dots". He said he didn't know how to play it optimally beyond 11 points, so I thought I'd have a go.

It goes like this:

Starting position: Player one marks a dot: which eliminates its neighbours: Player 2 marks another dot: leaving player one to take a dot: and win!

You have a row of dots, and you and your opponent take it in turns to mark one of them. The rule is that you can't mark a neighbour of an already marked dot, and if you thereby can't move, you lose.

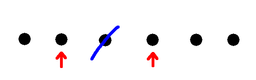
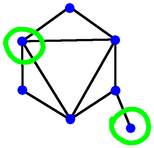
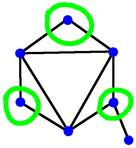
Here at right is an example game with 6 dots. Player 1 takes a dot somewhere in the middle, and that means now neither player can take the dot on either side. Player 2 removes the left-most dot, leaving the two on the far right.

Player 1 then wins by taking one of the remaining dots, which also eliminates the other, because it's a neighbour. So in this example game, Player 1 wins.

Now suppose we start with an odd number of dots. If Player one marks the middle dot, removing it and its neighbours from the game, we are left with dots on the left, and dots on the right, and no way of interacting. Now no matter what Player 2 does on one side, Player 1 can copy on the other. As a result Player 1 is guaranteed always to have a move, and therefore is guaranteed to win.

So with an odd number of dots, Player 1 can always win by marking the middle dot. (There might be other winning moves, but, well, who cares?)

So any row with an odd number of dots is a win for the first player.

Starting from scratch, a row with just 1 or 2 dots is a win for the first player, because they mark it, and that's the end. A row of 3 dots is a win for the first player if they choose the middle dot. If they are foolish enough to choose an end dot then the second player still has a place to play, takes it, and wins.

On the other hand, a row of 4 dots is a win for the second player, I'll leave it to the (mythical) interested reader to work that out, and to work out whether the 6 dot game is a win for the first or second player.

So how do we think about this?

First digression, a generalisation

You can skip this section if you want to - we're going to do a little Graph Theory.

A "Graph" or "Network" is a collection of nodes (also called vertices), some of which are joined by edges (also called lines).

Two vertices joined by an edge are called "Adjacent" or "Neighbours".

A set S of vertices is called "Independent" if it doesn't contain a pair of adjacent vertices. S is also called "Maximal" if every vertex is either already in the set, or has a neighbour who is. So once you have a maximal independent set of vertices in a graph, you can't add any more.

A graph		Not independent		Not maximally independent

Maximal independent

Maximal independent

And with all that, we get this: Rob's Dots game is "Monochrome" where the chosen graph is a path. I'm glad we got that settled. Moving on ...

Second digression, the game of Nim

Now let me introduce you, or perhaps re-introduce you, to the game of Nim. You may have met it before, but bear with me - this is relevant.

Very relevant.

In Nim we have some heaps of tokens - the heaps need not all be of the same size, and in general, aren't. On each move a player may take any number of items from any single heap, including the option of taking an entire heap. As with the dots game, if you can't make a move, then you lose.

The game of Nim has been solved. What does that mean? For a game to be solved means that given a game position there is a simple (or sometimes not so simple) calculation one can make to determine whether it is a win for player 1 or player 2. If it's a win for Player 1, and it's your turn, that means there is a winning move. So you then can look at all the positions you can move to and pick one that is a win for Player 2. Then do that.

There are some similarities here between Nim and the dots game. We can look at the collection of dots as starting as a single component, and then when you mark a dot, and thereby eliminate its neighbours, you've broken up the remaining dots into separate collections. We call them components, because when we think of this as an example of "Monochrome", taking vertices can separate the graph into separate pieces, and the separate pieces of graphs are called components.

So in the dots game, whenever there are two components of the same size (and more generally, in a game of Monochrome when there are two components that are isomorphic), whatever one player does in one, the other can mimic in the other, and so it's a second player win. Likewise in Nim, if there are two heaps of equal size then the second player can mimic the first player's moves and win.

So Nim has some similarities to the Dots game, and perhaps things we learn in the study of Nim can be of use in the study of the Dots game. And indeed, as it happens, both are examples of a more general type of game called an Octal Game[0].

Converting Dots to Heaps.

Since Nim is well studied and has lots of cousins, there might be value in thinking of the Dots game somehow as a collection of heaps. We do that as follows.

Given that we start with N dots, we think of that as equivalent to a heap with N tokens. When we mark a dot we remove it and any neighbours, and that might leave nothing, one path, or two paths.

So:

• If N=1 or N=2 then the only thing we can do is take the entire heap.

• If N=3 then we can take an endpoint, leaving one dot, or the middle dot, leaving nothing.
  • Thus if we have a heap of 3 we can:
    • take two items leaving 1, or
    • take all of it.

• If N=4 then we can take an endpoint, leaving two dots, or a "middle" dot, leaving 1 dot.
  • Thus if we have a heap of 4 we can:
    • take two items leaving 2, or
    • take 3, leaving 1.

• If N>=5 then we can:
  • Take two, leaving a single heap with N-2;
  • Take three, leaving a single heap with N-3;
  • Take three, and divide the remaining N-3 into two heaps.

This exactly falls into the class of Octal Games, and is given the octal encoding of 0.137. It's been studied a great deal, and is equivalent to Dawson's Chess[1], a simplified version of which is called Hexapawn[2], and was written about by Martin Gardner.

(This is one of the advantages of having a consistent encoding of games based on what the game is, rather than just a random name that someone made up. Calling the game "0.137" means we can find other games that are, underneath, the same).

An analysis of 0.137 ...

So we can now look at the octal game 0.137 and see what we can learn.

A little notation. We can describe a game position by listing the sizes of the heaps. Further, we can put the sizes in order. So an example position might be (2,4,7). The position with no heaps is (), and is a second person win, because the first player has no moves.

The notation P position means "Previous Player Win". The above comment about Nim being solved means that there is a simple way to determine whether a position is a P position or not, without having to go through the tedious process of investigating the tree of options. Positions that are not P positions are called N positions, for "Next Player Win".

We say that any position which is a second person win is a P position. Thus () is a P position. The earlier analysis also shows that (n,n) is a P position for any (positive integer) value n. It's also easy enough to verify that (4) is a P position.

We can also observe that if we have one P position, say (2,2), and another P position, say (4), then the combination of both sets of heaps, in this case (2,2,4), is also a P position. We capture this idea by saying that a P position is "decomposable" or "reducible" if it's a combination of two or more other, non-trivial P positions, otherwise it's "irreducible". So (2,2,4) is reducible.

Our challenge now is to find all the irreducible P positions for 0.137 ...

A list of P positions ...

So we already know that (), (4), and (n,n) for every integer value of n>0 are all P positions. What other P positions are there? If we write a computer program we can quickly check that these are the one-heap P positions up to 100:

• 4, 8, 14, 20, 24, 28, 34, 38, 42, 54, 58, 62, 72, 76, 88, 92, 96
  • (just writing the size of the heap)

P positions with 2 heaps, each heap <= 50

• (1,2), (1,6), (1,7), (2,6), (2,7), (6,7), (3,11), (5,9), (3,12), (5,10), (3,16), (9,10), (3,17), (1,21), (11,12), (1,22), (2,21), (5,18), (2,22), (5,19), (11,16), (1,26), (6,21), (9,18), (10,18), (11,17), (12,16), (1,27), (2,26), (3,25), (5,23), (6,22), (7,21), (9,19), (10,19), (12,17), (2,27), (7,22), (6,26), (9,23), (10,23), (16,17), (6,27), (7,26), (3,31), (7,27), (11,25), (1,35), (12,25), (1,36), (18,19), (2,35), (2,36), (3,37), (1,40), (16,25), (18,23), (6,35), (11,31), (13,29), (1,41), (17,25), (19,23), (2,40), (6,36), (7,35), (12,31), (21,22), (2,41), (7,36), (5,39), (15,30), (13,33), (6,40), (16,31), (21,26), (6,41), (7,40)

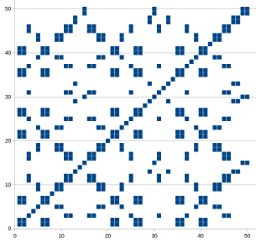
(These are ordered by the total number of tokens).

One thing we might ask is whether the density of P positions settles to some sort of limit. From the chart at right here the 2-heap P positions seem fairly uniformly spread. Does that pattern continue?

But here is a simple observation. A heap with one token is, as far as game play is concerned, exactly the same as a heap with two tokens. In each case the player would have to take the entire heap. So in this sense, 1=2.

That means that every time there is a P position with a 2 in it, we can replace the 2 with a 1. So we can see we have (1,6) and (2,6). We also have (1,7) and (2,7). And then we notice that we also have (6,7). So we start to wonder, if we have (a,b) and (b,c), do we then also have (a,c) ??

Actually, it appears that we do. We have (3,11) and (11,25), and yes, we also have (3,25). We also have (3,3), (11,11), (25,25), and so on. Perhaps we can, in some way, think of 3, 11, and 25 as being equivalent.

For example, although I haven't listed the triples here, there is a triple (2,10,17) and we also have (7,10,17). So remembering that (2,7) is a P position, we might hazard the guess that two numbers a and b such that (a,b) is a P position are, in terms of P positions, "equal" or "equivalent" to each other.

Looking at the pairings in two-heap P positions we find we get the following equivalence classes:

• 1, 2, 6, 7, 21, 22, 26, 27, 35, 36, 40, 41, 55, 56, 60, 61, 69, 70, 74, 75, 89, 90, 94, 95
• 3, 11, 12, 16, 17, 25, 31, 37, 45, 46, 51, 59, 71, 79, 80, 93
• 5, 9, 10, 18, 19, 23, 39, 43, 44, 52, 53, 57, 65, 73, 77, 78, 86, 87, 91
• 13, 29, 33, 47, 48, 63, 67, 81, 82
• 15, 30, 49, 50, 64, 83, 84
• 32, 66

Yes.

Excellent! Let's try another one. Again, let's find a 3 and replace it with (5,6). We look through and find that (3,7,9) is a P position, so we would hope that (5,6,7,9) would also be. But we look through our program output and don't find it. Has it gone wrong?

No, it hasn't, because (5,6,7,9) is reducible, being made up of (5,9) and (6,7). So it is a P position, and the idea has in fact worked in this example as well.

So whenever we have a triple tuple (a,b,c) it would appear that (a) is equivalent to (b,c), that (b) is equivalent to (a,c), and that (c) is equivalent to (a,b).

I wonder if that generalises ...

... and now a miracle occurs.

It will, by now, come as no surprise that yes, it generalises. But it not only generalises, it takes us into "This is too good to be true" territory, as a friend of mine calls it. Be warned, this next part takes us into some deep, technical stuff. But it's worth it - the pay-off is huge.

This is in contrast to a game such as chess, in which each player plays with their own pieces, and hence for any position the moves available to each player are different.

This game is an impartial game, meaning that from any given position each player has the same possible moves. You can see that for a given position in this game, the choices are the same, no matter whose move it is.

So here is the unbelievable result.

In a very real sense, which can be made technically precise:

The Sprague-Grundy theorem[6] states that every impartial game (with some reasonable limitations) is equivalent to a Nim heap of a certain size. The equivalence is not always simple or straight-forward, but it means that all games like this are more similar than otherwise might be expected. The technical condition is: A game is a two-player sequential game of perfect information in which there are no infinite lines of play and a player who cannot move loses.

• Any impartial game corresponds to a position in a game of Nim;
  • That's clearly a useful result, although a little hard to see why it's true.
• Any collection of Nim heaps corresponds to a single Nim heap;
  • That should cause one to raise an eyebrow - it seems vaguely implausible.
    • In particular, when combined with the previous point it means any impartial game corresponds to a single Nim heap.
• Any combination of positions from multiple impartial games all placed next to each other corresponds to a single Nim heap;
  • In this version you pick which game to play in when it's your turn
    • Well, now you're just being silly.

To understand how all this works we need to make precise the sense in which this game over here somehow corresponds to a single Nim heap, and then we need to work out what that implies. Here's how we start.

We are, for each size of heap in Rob's game, going to compute a thing called its "G-value". That's the size of a Nim heap that will, in some sense, be equivalent to the given heap in Rob's game.

In Rob's game having no points left to choose is, obviously, a P position. It corresponds to having no heaps left in a game of Nim. So the G-value of () is 0.

In each case of a heap of size 1 and a heap of size 2, the only thing you can do is move to (), and that's the same as the situation with a Nim heap of size 1. So the G-value of (1) is 1, as is the G-value of (2).

Starting from (3) we can move either to () or (1). So we can get to G-values of 0 or 1, and those are the options for a Nim heap of size 2. So the G-value of (3) is 2.

Here we are so far:

Rob's game heap size	Possible positions after a move	G-values after a move	G-value
0	None	-	0
1	()	0	1
2	()	0	1
3	(),(1)	0,1	2
4	(1),(2)	1,1	?

So what should the G-value of (4) be? We know it is a P position, so perhaps its G-value should be 0.

Yes.

Observation: we have not at this point explained why this is the definition, or why it works.

• Definition:
  • Consider the G-values of all the positions we can move to.
  • The G-value of this position is the smallest value that does not occur.

Rob's game heap size	Possible positions after a move	G-values after a move	G-value
4	(1),(2)	1,1	0

But what about the next position, (5), which has possible destination positions of (2), (3), and (1,1). We know the G-values for the first two of these - 1 and 2 respectively - but what about the G-value of (1,1)?

To take the XOR or Nim-sum of a collection of values, do this: Write them in binary; Align them; For each column put 1 if there are an odd number of 1's, 0 if there are an even number of 1's; Interpret the result as a binary number. Thus the Nim-sum of 1, 2, and 5 is: . 1 = 0 0 1 . 2 = 0 1 0 . 5 = 1 0 1 . ------- . 1 1 0 -> 6 So the Nim-sum of 1, 2, and 5, is 6.

And here is the second rule.

• Definition:
  • The G-value of a collection of G-values is the XOR, also known as the Nim-sum, of them all.

So the G-value of (1,1) is the XOR of the G-values of the components. The components are both (1), and so each component has G-value 1. So the G-value is 1 XOR 1, which is 0.

So our entry for 5 becomes:

Rob's game heap size	Possible positions after a move	G-values after a move	G-value
5	(2), (3), (1,1)	1,2,0	3

The G-value for (5) is 3, being the least value that doesn't appear in the third column.

And so we continue:

Rob's game heap size	Possible positions after a move	G-values after a move	G-value
0	None	-	0
1	()	0	1
2	()	0	1
3	(),(1)	0,1	2
4	(1),(2)	1,1	0
5	(2), (3), (1,1)	1,2,0	3
6	(3), (4), (1,2)	2,0,0	1
7	(4), (5), (1,3), (2,2)	0,3,3,0	1
8	(5), (6), (1,4), (2,3)	3,1,1,3	0
9	(6), (7), (1,5), (2,4), (3,3)	1,1,2,1,0	3
10	(7), (8), (1,6), (2,5), (3,4)	1,0,0,2,2	3
11	(8), (9), (1,7), (2,6), (3,5), (4,4)	0,3,0,0,1,0	2
12	(9), (10), (1,8), (2,7), (3,6), (4,5)	3,3,1,0,3,3	2

Looking at these we can list the sizes that all have the same G-value:

• 0: 0, 4, 8, ...
• 1: 1, 2, 6, 7, ...
• 2: 3, 11, 12, ...
• 3: 5, 9, 10, ...

Seriously, this looks really good. It is possible to look carefully at moves, Nim, games, sums of games, and derive the concept of the G-value, but those details are for another time. For now we need to take what we've done and see how to use it.

How to win at Rob's game

All of this is very interesting, but ultimately we want to win! So how can we turn this to our advantage?

The basic idea is this. Given a configuration, convert all heaps to their G-values, and then XOR them all together. This gives the G-value of the configuration. If the result is 0 then (with perfect play) it doesn't matter what we do, we have lost. It's a P position, and the previous player has won. So what we do is make the position as complex as possible to give our opponent the maximum opportunity for a mistake.

But what if the result is non-zero?

This is not straight-forward. The easiest thing to specify is simply to look at every possible move, compute the G-value of the result, and choose the one that gives an answer of 0. There will always be one. This process can be simplified, but it's messy.

So that just leaves the question of how to compute the G-values. We have a process outlined above - the smallest value that doesn't occur in the list of G-values of positions you can move to - but that's pretty tedious to work out, and it would be nice to have a simpler process.

There is.

It is a conjecture that for every game of this type, the sequence of G-values of larger and larger components is eventually periodic, and so it proves to be with this one. The G-value of a component of size n is given by:

• If n is 0, 14, or 34, then
  • the G-value is 0

• If n is 16, 17, 31, or 51, then
  • the G-value is 2.

• 8112031103322445593301130211045374

So here's how to win at Rob's Game.

• Think of the original dots as being points on a line;
• Marking a dot corresponds to deleting a dot and any neighbours;
• Look at each component, and count the number of dots in each;
• Compute the G-value for each component;
• XOR together all the G-values - that's the G-value of the current position;
• If that's 0, do anything you like;
• Otherwise:
  • For every move:
    • Look at the position you would get to;
    • If the resulting G-value is 0
      • Make that move.
  • This will eventually find a move that wins.

• If there's a component whose G-value is 0 then you can ignore it;
• If there are two components with the same G-value you can ignore them;
• If there's a sub-collection of heaps whose XOR of their G-values is 0, you can ignore them.
  • Note that this is a generalisation of the previous two cases.

And so on. But broadly, that's the entire game.

Solved.

A final example ...

A 50 dot game

So let's start with a game of 50 dots.

This has a G-value of 5, so there must be a winning move. We try taking an end-point, but g(48)=4, so that's no good. Try taking the second from end, but g(47)=4, so that's also no good. OK, we need to take an interior point.

Taking an interior point removes 3 from consideration, so we look at pairs of numbers that add up to 47 and see if we can find two that have the same G-value:

• (1,46) : g(1)=1, g(46)=2, nope;
• (2,45) : g(2)=1, g(45)=2, nope;
• (3,44) : g(3)=2, g(44)=3, nope;
• (4,43) : g(4)=0, g(43)=3, nope;
• (5,42) : g(5)=3, g(42)=0, nope;
• (6,41) : g(6)=1, g(41)=1, we have a winning move!

And so we continue. Suppose our opponent takes the middle point of the larger collection, leaving position (6,19,19).

What we do is observe that (19,19) is a P position, so if we can convert (6) into a P position, we're set. And we do that by taking an endpoint from the 6, leaving (4), which is a P position. So now we have two P positions, and we play each as a separate game. Whatever our opponent does, we do the right thing in that game, greatly simplifying the calculations we have to perform.

Addendum

Lest you think that everything is sorted, there is a book called "Unsolved problems in combinatorial games" by Richard J Nowakowski[7]. In that, question number 2 asks if all games such as we've been describing are, in fact, periodic.

We just don't know.

Take a specific example such as the game "Officers", which is 0.6 using the Octal Game notation[0]. We don't know if that's periodic, and as of November 2012, Achim Flammenkamp's list of octal games[8] has explored up to $2^{47}$ positions without a proven solution.

We just don't know.

So there are still problems to explore, results to be found, papers to be written, and games to be analysed. I hope this has been an interesting introduction to Combinatorial Game Theory[9].

Credits and References:

My thanks to Adam Atkinson, Adam Townsend, and Rob Eastaway of Maths Inspiration for extensive and detailed comments that have made this discussion infinitely better. My thanks also to Rob for introducing me to this intriguing game.

[0] https://en.wikipedia.org/wiki/Octal_game

[1] http://www.di.fc.ul.pt/~jpn/gv/dawsonchess.htm

[2] https://en.wikipedia.org/wiki/Hexapawn

[3] Mathematical Games, Scientific American, March 1962, reprinted in The Unexpected Hanging and Other Mathematical Diversions, by Martin Gardner, pp. 93ff

[4] https://en.wikipedia.org/wiki/Map-coloring_games#Monochrome_and_variants

[5] https://en.wikipedia.org/wiki/Octal_game#Game_specification

[6] https://en.wikipedia.org/wiki/Sprague-Grundy_theorem

[7] http://library.msri.org/books/Book63/files/131106-Nowakowski.pdf

[8] http://wwwhomes.uni-bielefeld.de/achim/octal.html

[9] https://en.wikipedia.org/wiki/Combinatorial_game_theory

<<<< Prev <<<< One Of My Favourite Puzzles

:

>>>> Next >>>> Disappearing Trains On Virgin ...

---

You should follow me on twitter

Send us a comment ...