
My latest posts can be found here: Previous blog posts:
Additionally, some earlier writings: |
Just teach my kid the <adjective> math
At face value, the strong reactions we see can be interpreted as paradoxical. Parents might openly admit they themselves did not understand mathematics, that they actively hate mathematics even, but insist that we don't dare do anything different for their child in math class! Parents' befuddlement over their child's third-grade homework might be seen as a wrong of the new curriculum, not as evidence of the failing of their own mathematics education, that they weren't provided the flexibility and agility of thought to see simple arithmetic in multiple lights. It seems that previous generations were seduced to equate familiarity with understanding. For instance, our standard arithmetic algorithms are somewhat bizarre - they are the end result of a human process of codifying arithmetical thinking, designed with the extra goal of using as little of precious 17th-century ink as possible. But if one does them often enough, their routine begins to feel comfortable and familiar. 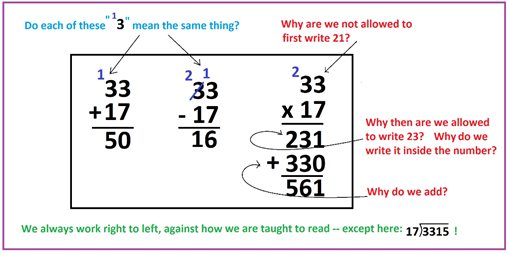 These algorithms are the comfort math of the generations of students for centuries past. And I would go further, these algorithms are the definition of mathematics for so many folk of the past. To not perform these procedures is to not do math. Reform is a threat to these algorithms and hence a threat to math, never mind that we're in the 21st-century when ink, paper, and slate chalk are no longer precious. And also never mind that we don't even need these algorithms - our smartphones will perform the paper-and-pencil computations with greater speed and greater accuracy than we humans. (I should make the point that despite these observations, the Common Core State Standards in the U.S. still requires student fluency with these algorithms: they continue to be taught. The Standards have simply delayed the introduction of these processes until the proper number sense and familiarity with arithmetic is in place so that these algorithms make sense and can be used in both routine and in flexible and clever ways. It is actually asking more of students with regard to these procedures than for past generations!) Nonetheless, the potent reaction to math change speaks a truth. It shows that our society feels (or is it understands?) that mathematics is a vital and core human enterprise, that it offers power for operating in the world, and that deprivation of effective mathematics learning in one's childhood will likely severely hinder success in adult life. Why else does change to mathematics teaching elicit emotional outrage? Parents may feel they have "missed out" on something and, rightly, do not want their children to possibly miss out too. I fully agree with the sentiment of the parental outrage given this interpretation. So ... Can we educators work to understand this sentiment, put the right words to it, and fully engage in conversation about it? Can we be fully transparent about our approaches and intents and listen to, honor, learn from, and respond to parental and societal reaction with clarity and grace? Of course we can and of course we must. Consider this example of "new math" absurdity that made the internet rounds a few years ago. We can learn from it. Even if this is a false write-up of some supposed classroom work, someone went to the effort to write and post this piece to make some points. We should hear what is being said: it illustrates some failings we educators can easily fall into, and sometimes do. 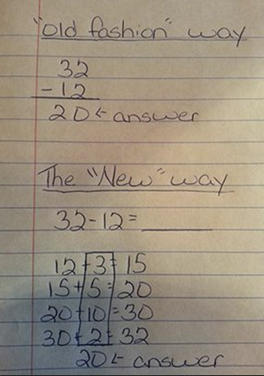
Our lesson: We need to communicate the true goal of given exercises to parents. 2. By writing 32-12 = 20 at the top of the page, the author is likely also making that point that the arithmetic here is straightforward: one can just see the answer is 20! The convoluted approach at the bottom of the page is thus an absurd manner for answering the problem. And the author is right! Our lesson: If we are going to ask students to practice mathematics ideas, we need provide interesting or meaningful examples with which to practice them. An example like 73-57 or 2018-1995 might be better.
Our lesson: We need to be sure not to insist on one approach when analyzing a problem. We need to encourage students to generate efficient practices. If the point of using such a straightforward example was simply to develop fluency in this anchoring technique, then we need to make clear to student and parent alike that this work is simply practice work and not couch it in a framework that suggests it is the required arithmetical approach. 3. The student part of the piece is written with pedantic care. Perhaps the author here is making the point that he thinks students are always required to undertake the arduous task of showing his or her work no matter how tedious that enterprise might be. Parents are right to react to this when it is indeed laborious and mind-numbing. Our lesson: Work to have students show their work only when there is work worth showing. Let's honor our students' intellectual capabilities and time! Sure, there is pedagogical care to be undertaken here, that students need to practice explaining mathematical thinking with (meaningful!) beginning examples, but it need not be every example, each and every time. Our lesson: Make showing/explaining your work interesting. So often we couch a mathematics task as simply to get the right arithmetical answer. If, for example, one can just see that the answer is 20, then there is no point going to any more effort than just stating that the answer is 20! So let's not ask just computational questions, but also ask direct thinking questions with the computational answers already provided:
Our lesson: Mathematics education is changing: it is working to couple understanding with all the same mathematics that was studied in the past. We need to acknowledge that there is in fact a change. Fear of change can manifest itself in all sorts of curious ways. One parent argued "You don't need to understand how a car works in order to drive one" and another demanded "Just teach my kid the <expletive> math," wanting a procedure demonstrated, not explained. The 21st-century is not looking for humans who serve as calculating machines, but instead it seeks problem-solvers and innovative thinkers. Google, Adobe, Apple and NASA need people who not only know the standard procedures of mathematics, but can also think with agility, flexibility, and innovation. They want people who have the confidence to create new ideas and explore them, tweak them, and find new paths of innovation never conceived of before. Mathematics can, and should, train for such innovative thinking. And this leads to my final point. 5. If we truly acknowledge there is a change in mathematics education - as this internet piece purports to demonstrate - then we need to stand by what we value: understanding, flexibility of thought, innovation, problem-solving, reflection on solutions and approaches, and the search for efficiency and elegance. Our lesson: We need to assess what we value and precisely what we value. If all our assessments are about computation with speed, for which memorization without understanding is a good strategy for surviving, then we are hypocrites. Let's be honest and true to our goals, even with testing. Sadly, this is where the fear of parents is absolutely valid: in the high-stakes testing of college admissions we educators have no right to "mess with" students' chances of receiving high standardized test-scores. Our lesson: We must acknowledge that testing agencies in most parts of the world have not yet caught up with what we educators value. We must find the means for students to experience tremendous success on all fronts - with speed testing and with deep understanding and mathematical innovation. We can assess and reward the latter in our classrooms at least. So let's do so! And we can couple memorization and fluency of procedure with understanding. So let's do that too! And we can do all this with communicated clarity and transparency, with flexibility, and with inclusive conversation. With potent emotional reactions it is clear that parents are fully invested in supporting K-12 mathematics learning. They are "all in." Think how terrific that is! What a tremendous opportunity we have to harness and clarify the emotion and foster powerful united support for our students' education! James Tanton - the authorBringing joyful, genuine, meaningful, uplifting learning to the world is my thing ... especially with mathematics. Global Math Project, Beagle Learning & more!
Send us a comment ...
|

 Suggest a change ( <--
What does this mean?) /
Send me email
Suggest a change ( <--
What does this mean?) /
Send me email