This page is an outline of the Juggling Talk that I give. It is not
intended to be complete, but merely to give a flavour of just some
of the ideas covered.
Juggling - Theory and Practice.
Introduction
In 1985 there arose, simultaneously in three places around the
world, by groups entirely unconnected and completely ignorant of each
others' existence, a notation for juggling tricks. The notation was
incomplete, since not every trick could be described, and like many
notations, it was not immediately apparent to the uninitiated how to
read it, how to use it, or whether it would be of any real use. For
those who understood it, however, it was instantly obvious that it was
right. Somehow the notation managed to capture the essence of
those tricks it described, and the fact that the same notation arose in
more than one place at once showed that its time had come, and it was,
quite simply, the notation.
Since then the notation, now known as Site Swap notation, has become
fairly well-known in juggling circles. Reactions were initially somewhat
mixed. In juggling, as with music, there are those who study the works
produced by others, there are those who produce their own, and there are
those, the juggling equivalent of the jazz musician, who feel that while
it may be of use to some, juggling has a soul, and should not be trapped,
caged, and prevented from varying from one, single form. Over time,
however, the notation has gained acceptance by the majority, and it is
now considered a useful tool for the communication of tricks, incomplete
though it is.
First steps - make juggling simpler
It is impossible to show in written form the infinite variety of
juggling tricks that can be performed. Some have the arms moving
sinuously past each other, somehow managing to toss, catch and carry
three balls, never more than one per hand at a time, always moving over
and past each other. Others have the hands largely stationary with the
balls, rings, clubs, fire-torches or chainsaws spinning to various
heights, seemingly none the same. Such variety can never fully be
captured, and there is always room for the performer's own
interpretation of the basic moves, the underlying patterns. The Site Swap
notation describes the trick that is the basis on which variations can
then be built.
To make precise the limitations we place on ourselves, we state a
specific set of rules that must be obeyed. These rules seem terribly
restrictive, but within the resultant framework we will find that there
is structure that can be exploited. The rules we use are as follows:
- We only juggle balls.
- Only one ball is thrown or caught at a time.
- The hands stay on their own side of the body.
- Throws happen inside shoulder width.
- Catches happen outside shoulder width.
- Throws and catches happen at a fixed speed.
- The hands alternate.
- The hands are full for half the time.
We will not attempt here to explain or justify these rules. Instead we
will describe the practical results, and show why they are of interest
both to jugglers and to mathematicians.
Investigating the consequences
To investigate the consequences of the rules, let's look at juggling
three balls, and let's start with a further simplification. For now,
let's assume for now that we not only obey the rules, but also insist
that every throw must be identical.
Some quick experimentation soon shows that this then forces each
ball to be thrown in sequence, for otherwise there would be variations
in the timings. We start by throwing ball A with the right hand, then
ball B with the left, then ball C with the right. Now we have to make a
throw with the left hand and it must be ball A. Each ball is thrown
every third throw, and from this we can conclude three things. The first
is that it will work, the second is that the balls don't go in the
expected "Big Circle" seen on every popular picture of a clown juggling,
and the third inescapable conclusion is that the balls are forced to
change hands.
Do the same thing with four balls and we discover now that the balls
cannot change hands. If we juggle four balls with every throw the same,
and subject to our rules, we end up juggling two balls in each hand,
independently, but asynchronously. There is no alternative, and in the
live version of this presentation, whether it is to adults or to
children (generally 14 or older), to scientists, mathematicians or lay
people, the audience can see this for themselves.
With five balls they once again cross, with six balls again it
becomes half in each hand. These are unavoidable consequences of our
rules. With each ball being thrown in exactly the same way, each ball is
forced to take it's turn. With an odd number, that means that each ball
will alternate hands, but with an even, each ball will have to come back
to the same hand.
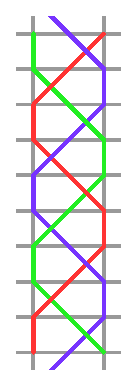
3 ball cascade
Figure 1
|
To make it easier to examine what's happening in a
juggling pattern we make use of a diagram such as Figure
1. In this diagram the vertical grey lines represent the
hands, the horizontal grey lines show the beats of the
music to which we are juggling, and the coloured lines
bouncing back and forth between the hands represent the
balls. Figure 1 shows the three ball cascade, the
pattern with three balls in which every throw is the
same.
We can see clearly now how the balls weave around each
other. Indeed, if a ribbon is attached to each ball, and
the other ends of the ribbons held some distance away,
juggling this pattern will plait the balls.
Diagrams such as Figure 1 are called Space-Time
Diagrams because they show both space and time on the
same chart. Jugglers will often call it a Ladder Diagram,
for obvious reasons.
|
If we look at how long each ball spends in the air, an
obvious pattern emerges. With three balls, each ball is
thrown every third throw, and since it spends one beat
of time in the hand it must therefore spend two beats of
time in the air. Similar reasoning gives us the
following table:
Number
of balls |
Beats between
throws |
Time in
the air |
| 3 | 3 | 2 |
| 4 | 4 | 3 |
| 5 | 5 | 4 |
| 6 | 6 | 5 |
| 7 | 7 | 6 |
| ... and so on ... |
The central column here is called the "Cycle Time," and
turns out to be the single most important idea in the
development of the theory of juggling.
From this table we can, should we choose, deduce what
the physical throw heights must be and derive formulae
for how hard we must throw. This, however, is not the
most interesting direction to take.
Generalising slightly
Consider now what happens if we are juggling four balls
in the pattern where all of the throws are the same.
Every ball goes into the air, comes down, gets held in
the hand, and then goes off into the air again. If any
single throw is too high, or too low, the pattern is
ruined.
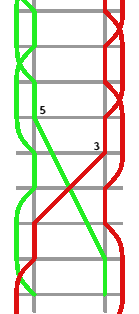
Figure 2
|
However, if one single throw is made as if for one
instant we were juggling five, and the very next throw
is made as if we were juggling only three, what happens?
The first throw is higher, stays in the air for a beat
longer, and comes down in the other hand. We would
expect it to clash with another ball landing at that
time in that hand, but that has been preempted. The ball
with which it would have collided is the very ball that
has been thrown low and to the other hand. The timing
has conspired to allow the two balls to exchange places
in the pattern. They have swapped their landing sites,
and hence the name for the notation, Site Swap.
This rather unlikely pattern is annotated by writing
down the cycle times of the throws involved:
... 4 4 4 5 3 4 4 4 ..., showing that in the middle of
an infinite sequence of throws with cycle time "4", we
do a "5" followed immediately by a "3".
|
Click SS:44445344 to see this trick.
There are other possibilities too. Here is a table of such
sequences.
a: ... 4 4 4 4 4 4 4 ...
b: ... 4 4 4 5 3 4 4 4 ...
c: ... 4 4 4 5 5 2 4 4 4 ...
d: ... 4 4 4 5 5 5 1 4 4 4 ...
The fourth of these sequences,
... 4 4 4 5 5 5 1 4 4 4 ... was, incidentally,
discovered by writing down exactly this sequence of
sequences. The first three were already well-known to
the juggling community, and given the clear and obvious
progression, the fourth really ought to be a juggling
trick, and it is.
But what does it mean to have a cycle time of 1? By
referring to our table above we can see that the air
time is always one less, so that implies that a throw
with a cycle time of "1" should have an air time of "0".
Does that make sense?
Yes it does. Try to "juggle" ("manipulate" might be a
better word) only one ball so that each hand is full for
exactly half the time. The ball must effectively
teleport between hands, and we do this simply by passing
the ball from one hand to another. We cheat slightly,
technically we are breaking rule 6, but in principle it
can (almost) be done.
What does pattern "d" look like? In the middle of
juggling two balls in each hand we must suddenly launch
one high and crossing, and the next high and crossing,
and the next high and crossing. For a moment we are
effectively juggling 5 balls, but it can't go on. We
only have one ball left, and we want to make two more
throws. So we cheat, and slide the remaining ball
directly into the other hand. The other three come down,
and we are once again juggling 4 balls in the standard
pattern.
You can click on these links to see this trick:
Predicting the future
What about the obvious missing sequence above, why stop
at sequence "d"? Surely we should be able to juggle
... 4 4 4 5 5 5 5 0 4 4 4 ... ??
Pause for thought: what does a cycle time of 0 imply? It
requires that the ball have an air-time that is one
less, which is -1. It would require that when the cycle
time 0 throw is made, the ball must travel backwards in
time for one beat.
Unbelievably, this can be done! There are three
interpretations. One is purely practical, and the other
two are straight out of the fairy-tale world of quantum
physics.
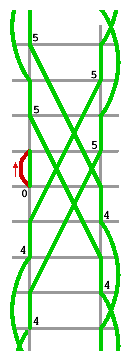
Figure 3
|
The most theoretically satisfying explanation is that the
ball really does go backwards in time. During the live
version of this presentation we will have met the idea of
a space-time diagram and used it in depth to analyse
several juggling tricks. The most obvious space-time
diagram for the sequence ... 4 4 4 5 5 5 5 0 4 4 4 ...
is shown in Figure 3.
As we can clearly see, the cycle-time 0 throw goes
backwards! Of course, many would argue that this simply
isn't physically possible, and in some senses they would
be right, but there's more going on here than that.
|
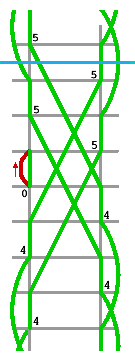
Figure 4a
|
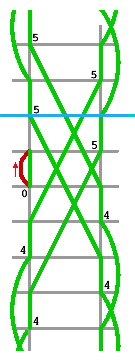
Figure 4b
|
How would a flash from a camera be represented on the
space-time diagram? It would be everywhere in space, but
only occur at one point in time. It would therefore be a
horizontal line on our diagram, and by seeing where the
trace from each ball crosses that line we can see where
each ball would be in the photograph. In Figure 4a we
have drawn a horizontal line at the time of a flash, and
we can see that there's a ball in the right hand, a ball
in the air above the right hand, a ball coming in to the
left hand, and the first of our high throws has just
left the left hand on its way to the right hand. In
Figure 4b there is a high throw just leaving the left
hand, a ball that's just landed in the right hand, and
two high in the air crossing from hand to hand.
|
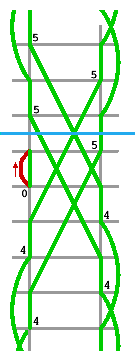
Figure 4c
|
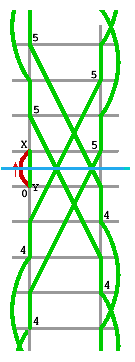
Figure 4d
|
In Figure 4c we have two high in the air crossing over,
high throw number three has just left the left hand, and
the right hand is holding the last ball to be thrown. In
each case we have four balls.
What about Figure 4d ??
There are four balls high in the air crossing over, and
one ball in the left hand. That makes a total of five
balls so far when we are only juggling four!
|
The other ball, the one travelling backwards in time,
must therefore be a negative ball. This is exactly
what quantum physics says. In all the quantum physics
equations an anti-particle is identical to a particle
travelling backwards in time. A positron is simply an
electron going backwards in time, a proton is an
anti-proton going backwards in time, and a photon is its
own anti-particle so it doesn't know if it's coming or
going, and that's closely related to why it travels at
the speed of light, and that ties in with the Special
Theory of Relativity. So what we have at time X is the
mutual creation of a ball/anti-ball pair, and at time Y
we have the mutual annihilation of the same pair. [See
footnote 2]
Interpreting the model
Of course the truth is much, much simpler.
The rules we laid down gave us a model of juggling,
and we have been working inside the model. Until now it
has agreed closely with reality, but we have finally
broken some of the rules. We are juggling four balls and
have made four high throws. There are no balls left, and
so we cannot have our hands full for half of the time.
The theory insists on it, and thus creates this
anti-ball, but the reality is that for one beat we have
an empty hand. What else can juggling 0 balls be but
standing there with empty hands.
We can use this to draw a clear distinction between
models and reality, and to show that an inaccurate model
is not a barrier to usefulness. Provided we understand
the assumptions on which our models are based, and
recognise the inappropriate predictions caused by our
stepping outside them, the models can still be useful.
As a case in point we have used our model to create a
method of inventing juggling tricks. Not all sequences
of numbers are valid, legal juggling tricks. This can
form the basis of an investigation: which sequences
work, which ones don't, and why? Using the space-time
diagram can assist in the understanding of the ideas,
but using an animation package helps even more.
Here is one way of inventing juggling tricks. It is
guaranteed to create sequences that work.
| Write down any set of consecutive numbers: |
| Now write them down in any other ordering: |
| Take the number of balls to be juggled: |
|
| 5 | 6 | 7 | 8 | 9 |
| 6 | 8 | 5 | 9 | 7 |
| 3 | 3 | 3 | 3 | 3 |
|
| Juggling trick = Row 3 plus row 2 minus row 1: |
|
|
There are several things to spot about this table. Just
one example: the numbers in the final sequence always
average to the number of balls in the juggling trick. It
works even if you start with negative numbers.
The real questions are:
- How does it work?
- Will it always work?
- Can we get every possible valid trick?
The answers are yes, yes and not really, but sort-of. We'll
leave you to explore why those are the right answers.
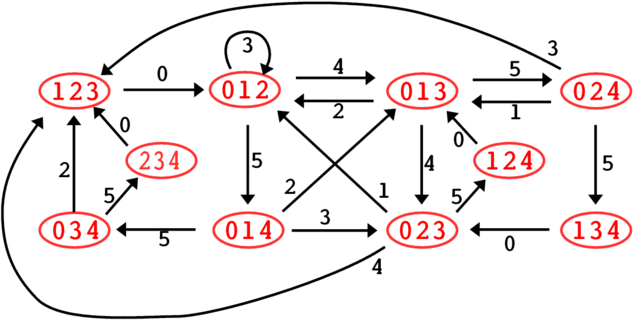
Here's another way to invent juggling sequences.
Start anywhere, follow the arrows, write down the black
numbers as you go, and finish where you started. The result
is always a juggling sequence.
Challenge questions:
- There are sequences that can be created using
this second process that cannot be created
using the table method.
- What are they?
- Why are the predictions different?
- Which one is better?
- There is an arrow missing. What is it?
- How was this diagram created?
Conclusion:
In closing, it's worth reviewing the processes that take
place during the presentation. Most of the audience
cannot juggle, and will never have taken a close look at
juggling, and yet along the way they deduce that the
balls must cross when there's an odd number of them in
the standard pattern, and cannot cross when there's an
even number in the standard pattern. They deduce the
consequences of the rules, use them to invent juggling
tricks, they see what happens when the model is pushed
too far. They observe, form hypotheses and test them. In
short, they are following the idealised scientific
process. By three-quarters of the way through they have
seen the distinction between models and the reality they
represent, recognising that models are intended to help
us understand the reality. They have also have seen what
happens when things go wrong!
Towards the end of the presentation I generally find
that several of the audience are asking "Why?" and "Does
it always work?" which gives me the ideal opportunity
to investigate justification and its close friend -
proof. The power of a proof is that it can show that
something must happen, can never go wrong, no matter
how many examples you try. It lets me draw the
distinction between proofs that are enough for everyday
use and proofs that allow for the most bizarre
situations. Thus we can see how mathematical proofs
relate to everyday proofs; their similarities and their
differences.
In short, this presentation gives literally dozens of
starting points for investigations, proofs, projects and
understanding. More than that, it shows that mathematics
isn't just arithmetic, and isn't only in the classroom.
It emerges in the most unlikely places.
Juggling, for example.
(c) Colin Wright,
2000, 2007.
Footnote 1: The Juggling Information Service can be
found at http://www.juggling.org and contains a very
large repository of information about juggling and
related material. Unfortunately it is not currently
being maintained, but one can find several juggling
animation packages there. Another resource is here:
The author's own package, Juggle Krazy, is available,
as is a description of the Site Swap notation aimed at
jugglers.
Footnote 2: The purists will argue that the creation
of a ball/anti-ball pair must require enormous amounts
of energy. However, just as there is an uncertainty
principle linking momentum and position, so there is
an uncertainty principle linking energy and time. Since
we are assuming that the throws and catches occur at
specific, and therefore known, times, we cannot know how
much energy is involved. Therefore we can borrow from
the quantum uncertainty for energy and create a virtual
juggling ball/anti-ball pair, provided we have them
annihilate within an appropriate time frame. The
anti-ball cannot exist for very long. This is a direct
analogy with the quantum vacuum.
Contents
| |
Links on this page
| |
Site hosted by
Colin and
Rachel Wright:
- Maths, Design, Juggling, Computing,
- Embroidery, Proof-reading,
- and other clever stuff.
|
|
 Suggest a change ( <--
What does this mean?) /
Send me email
Suggest a change ( <--
What does this mean?) /
Send me email
Front Page /
All pages by date /
Site overview /
Top of page







 Suggest a change ( <--
What does this mean?) /
Send me email
Suggest a change ( <--
What does this mean?) /
Send me email